Centre of mass and centre of curvature

This demonstration shows the relationships between the three states of equilibrium and three relative locations of the centres of mass to the centres of curvature of bodies.

    
a.                                            b.
Figure 2.A1: The models

Figure 2.A1 shows four small aluminum axially symmetric objects that have different dimensions and different relative locations of their centres of mass to the centres of curvature (The centre of curvature of a curve at any point is the centre of the circle which is tangent line at the point on the curve. If a line is drawn perpendicular to the curve at the point, the intersection point of the line and the vertical axis of symmetry is the centre of curvature):

    a. A round ball: The centre of mass and the centre of curvature of the ball are at the same point, the centre of the ball.

    b. A circular solid cylinder attached to a small part of a solid sphere: The centre of mass of the object is lower than the centre of curvature at any point on the spherical surface.

    c. A circular solid cylinder attached to a half of a solid sphere: The centre of mass of the object is higher than the centre of curvature at any point on the spherical surface.

    d. A hollow circular cylinder attached to a small part of a solid sphere: The centre of mass of the object is lower than the centre of curvature at any point on the spherical surface.

An experiment may be conducted as follows:

    a. Applying a small lateral force on the ball causes it move from its original equilibrium position (Figure 2.A2a). It moves to a new position and a new state of equilibrium (Figure 2.A2b). The original state of equilibrium is a position of neutral equilibrium.

    
a. Initial position of the ball                       b. New position of the ball
Figure 2.A2: Neutral equilibrium

    b. Applying a lateral force on the top of the object (b) rotates the object as shown in Figure 2.A3a. Releasing the force, the object returns to its original equilibrium position (Figure 2A3b). The original state of equilibrium is a position of stable equilibrium.

    
a. Object (b) with applied lateral force                       b. Object (b) returns to its original position
Figure 2.A3: Stable equilibrium

    c. Allying a force on the top as above but object (c) of the third object and holding it to the position from its original equilibrium position as shown in Figure 2.A4a. When the finger is removed the object falls over as shown in Figure 2.A4b. This original state of equilibrium is a position of unstable equilibrium.

    
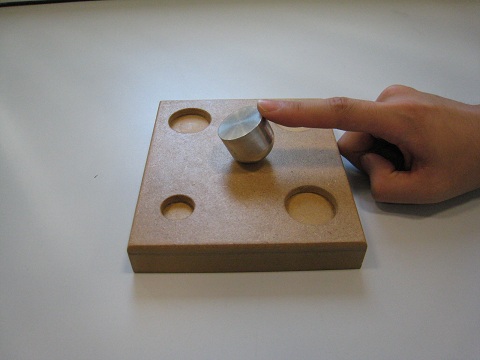
a. Object (c) with applied lateral force                       b. Object (b) Object (c) topples over
Figure 2.A4: Unstable equilibrium

    d. Applying a lateral force on the top of object (d) to the position shown in Figure 2.A5a. When the force is removed, the object moves back to its original position. The original state of a position of stable equilibrium.

    
a. Object (d) with applied lateral force                       b. Object (d) returns to its original position
Figure 2.A5: Stable equilibrium

It is observed from this demonstration that the states of equilibrium relate to the relative positions of the centres of mass to the centres of curvature of bodies:

• If the centre of mass and the centre of curvature of a body are at the same point, the body is in a state of neutral equilibrium.
• If the centre of mass is lower than the centre of curvature of a body, the body is in a state of stable equilibrium.
• If the centre of mass is higher than the centre of curvature of a body, the body is in a state of instable equilibrium.